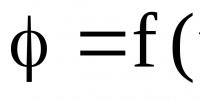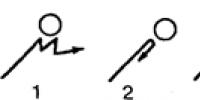The motion of a body along an inclined plane is a classic example of the motion of a body under the action of several non-co-directional forces. The standard method for solving problems of this kind of motion is to expand the vectors of all forces into components directed along the coordinate axes. Such components are linearly independent. This allows one to write down Newton's second law for the components along each axis separately. Thus, Newton's second law, which is a vector equation, turns into a system of two (three for a three-dimensional case) algebraic equations.

The forces acting on the block
case of accelerated downward movement

Consider a body that is sliding down an inclined plane. In this case, the following forces act on it:

• Gravity m g , directed vertically down;
• Support reaction force N , directed perpendicular to the plane;
• sliding friction force F tr, directed opposite to the speed (up along the inclined plane when the body slips)

When solving problems in which an inclined plane appears, it is often convenient to introduce an inclined coordinate system, the OX axis of which is directed downward along the plane. This is convenient, because in this case only one vector will have to be decomposed into components - the vector of gravity m g , and the friction force vectors F tr and support reaction forces N already directed along the axes. With this expansion, the x-component of gravity is equal to mg sin( α ) and corresponds to the "pulling force" responsible for the accelerated downward movement, and the y-component - mg cos( α ) = N balances the reaction force of the support, since there is no body movement along the OY axis.
sliding friction force F tr = µN proportional to the reaction force of the support. This allows us to obtain the following expression for the friction force: F tr = mmg cos( α ). This force is opposite to the "pulling" component of gravity. Therefore, for body sliding down , we obtain the expressions for the total resultant force and acceleration:

F x= mg(sin( α ) – µ cos( α ));
a x= g(sin( α ) – µ cos( α )).

It is not difficult to see that if µ < tg(α ), then the expression has a positive sign and we are dealing with a uniformly accelerated movement down the inclined plane. If µ >tg( α ), then the acceleration will have a negative sign and the motion will be equally slow. Such movement is possible only if the body is given an initial velocity down the slope. In this case, the body will gradually stop. If, subject to µ >tg( α ) the object is initially at rest, then it will not begin to slide down. Here, the static friction force will fully compensate for the "pulling" component of gravity.

When the coefficient of friction is exactly equal to the tangent of the angle of inclination of the plane: µ = tg( α ), we are dealing with the mutual compensation of all three forces. In this case, according to Newton's first law, the body can either be at rest or move at a constant speed (In this case, uniform motion is only possible downwards).

The forces acting on the block
sliding on an inclined plane:
up slow motion case

However, the body can also drive up the inclined plane. An example of such a movement is the movement of a hockey puck up an ice slide. When a body moves upward, both the friction force and the "pulling" component of gravity are directed downward along an inclined plane. In this case, we are always dealing with equally slow motion, since the total force is directed in the direction opposite to the speed. The expression for the acceleration for this situation is obtained in a similar way and differs only in sign. So for body sliding up an inclined plane , we have.

An inclined plane is a flat surface at some angle to the horizontal. It allows you to lift the load with less force than if this load was lifted vertically upwards. On an inclined plane, the load rises along this plane. At the same time, he overcomes a greater distance than if he rose vertically.

Note 1

Moreover, how many times there is a gain in strength, so many times the distance that the load will overcome will be greater.

Figure 1. Inclined plane

If the height to which the load must be lifted is equal to $h$, and thus the force $F_h$ would be expended, and the length of the inclined plane is $l$, and the force $F_l$ is expended, then $l$ is related to $h $ as $F_h$ is related to $F_l$: $l/h = F_h/F_l$... However, $F_h$ is the weight of the load ($P$). Therefore, it is usually written as follows: $l/h = P/F$, where $F$ is the force lifting the load.

The amount of force $F$ that must be applied to a load of weight $P$ in order for the body to be in equilibrium on an inclined plane is equal to $F_1 = P_h/l = Psin(\mathbf \alpha )$ if the force $P$ is applied parallel to the inclined plane plane (Fig.2, a), and $F_2$ = $Р_h/l = Рtg(\mathbf \alpha )$, if the $Р$ force is applied parallel to the base of the inclined plane (Fig.2, b).

Figure 2. Load movement on an inclined plane

a) the force is parallel to the plane b) the force is parallel to the base

The inclined plane gives a gain in strength, with its help it is easier to lift the load to a height. The smaller the angle $\alpha $, the greater the gain in strength. If the angle $\alpha $ is less than the angle of friction, then the load will not move spontaneously, and an effort is needed to pull it down.

If we take into account the friction forces between the load and the inclined plane, then the following values ​​are obtained for $F_1$ and $F_2$: $F_1=Рsin($$(\mathbf \alpha )$$\pm$$(\mathbf \varphi )$) /cos$(\mathbf \varphi )$; $F_2=Рtg($$(\mathbf \alpha )$$\pm$$(\mathbf \varphi )$)

The plus sign refers to moving up, the minus sign to lowering the load. Inclined plane efficiency $(\mathbf \eta )$1=sin$(\mathbf \alpha )$cos$(\mathbf \alpha )$/sin($(\mathbf \alpha )$+$(\mathbf \varphi )$) if the force $P$ is directed parallel to the plane, and $(\mathbf \eta )$2=tg$(\mathbf \alpha )$/tg($(\mathbf \alpha )$+$(\mathbf \varphi )$) if the force $P$ is directed parallel to the base of the inclined plane.

The inclined plane obeys the "golden rule of mechanics". The smaller the angle between the surface and the inclined plane (i.e., the flatter it is, not rising steeply), the less force must be applied to lift the load, but the greater the distance will need to be overcome.

In the absence of friction forces, the gain in force is $K = P/F = 1/sin$$\alpha = l/h$. In real conditions, due to the action of the friction force, the efficiency of the inclined plane is less than 1, the gain in force is less than the ratio $l/h$.

Example 1

A load weighing 40 kg is lifted along an inclined plane to a height of 10 m while applying a force of 200 N (Fig. 3). What is the length of the inclined plane? Ignore friction.

$(\mathbf \eta )$ = 1

When a body moves along an inclined plane, the ratio of the applied force to the weight of the body is equal to the ratio of the length of the inclined plane to its height: $\frac(F)(P)=\frac(l)(h)=\frac(1)((sin (\ mathbf \alpha )\ ))$. Hence $l=\frac(Fh)(mg)=\ \frac(200\cdot 10)(40\cdot 9,8)=5,1\m$.

Answer: The length of the inclined plane is 5.1 m

Example 2

Two bodies with masses $m_1$ = 10 g and $m_2$ = 15 g are connected by a thread thrown over a fixed block installed on an inclined plane (Fig. 4). The plane forms an angle $\alpha $ = 30$()^\circ$ with the horizon. Find the acceleration with which these bodies will move.

$(\mathbf \alpha )$ = 30 degrees

$g$ = 9.8 $m/s_2$

Let us direct the OX axis along the inclined plane, and the OY axis perpendicular to it, and project the vectors $\ (\overrightarrow(Р))_1\ and\ (\overrightarrow(Р))_2$ onto these axes. As can be seen from the figure, the resultant of the forces applied to each of the bodies is equal to the difference between the projections of the vectors $\ (\overrightarrow(P))_1\ and\ (\overrightarrow(P))_2$ onto the OX axis:

\[\left|\overrightarrow(R)\right|=\left|P_(2x)-P_(1x)\right|=\left|m_2g(sin \alpha \ )-m_1g(sin \alpha \ )\right |=g(sin \alpha \left|m_2-m_1\right|\ )\] \[\left|\overrightarrow(R)\right|=9.8\cdot (sin 30()^\circ \ )\cdot \ left|0.015-0.01\right|=0.0245\ H\] \

Answer: Accelerations of bodies $a_1=2.45\frac(m)(s^2);\ \ \ \ \ \ a_2=1.63\ m/s^2$

In addition to the lever and the block, simple mechanisms also include an inclined plane and its varieties: a wedge and a screw.

INCLINED PLANE

An inclined plane is used to move heavy objects to a higher level without directly lifting them.
Such devices include ramps, escalators, conventional stairs and conveyors.

If you need to lift the load to a height, it is always easier to use a gentle slope than a steep one. Moreover, the lower the slope, the easier it is to do this work. When time and distance are not important, but it is important to lift the load with the least effort, the inclined plane is indispensable.

These drawings can help explain how the simple TILT PLANE mechanism works.
Classical calculations of the action of an inclined plane and other simple mechanisms belong to the outstanding ancient mechanic Archimedes of Syracuse.

During the construction of temples, the Egyptians transported, raised and installed colossal obelisks and statues, the weight of which was tens and hundreds of tons! All this could be done using, among other simple mechanisms, an inclined plane.

The main lifting device of the Egyptians was an inclined plane - a ramp. The frame of the ramp, that is, its sides and partitions. As the pyramid grew, the ramp was built on. Stones were dragged along these ramps on sleds. The ramp angle was very slight - 5 or 6 degrees.

Columns of the ancient Egyptian temple in Thebes.

Each of these huge columns was dragged by slaves along a ramp - an inclined plane. When the column crawled into the pit, sand was raked out through the hole, and then the brick wall was dismantled and the embankment was removed. Thus, for example, the sloping road to the pyramid of Khafre, with a height of 46 meters, had a length of about half a kilometer.

A body on an inclined plane is held by a force that is as many times smaller than the weight of this body as the length of the inclined plane is greater than its height.
This condition for the balance of forces on an inclined plane was formulated by the Dutch scientist Simon Stevin (1548-1620).

Drawing on the title page of the book by S. Stevin, with which he confirms his wording.

The inclined plane at the Krasnoyarsk hydroelectric power station is very ingeniously used. Here, instead of locks, there is a ship's chamber moving along an inclined overpass. For its movement, a traction force of 4000 kN is required.

And why do mountain roads wind in a gentle "serpentine"?

A wedge is a variation of a simple mechanism called an "inclined plane". The wedge consists of two inclined planes, the bases of which are in contact. It is used to obtain a gain in strength, that is, with the help of a smaller force to counteract a larger force.

When chopping firewood, to facilitate the work, a metal wedge is inserted into the crack of the log and beaten on it with the butt of an ax.

The ideal gain in strength given by the wedge is equal to the ratio of its length to the thickness at the blunt end. Due to the high friction, its efficiency is so small that the ideal gain does not really matter.

Another type of inclined plane is the screw.
A screw is an inclined plane wound around an axis. The thread of a screw is an inclined plane repeatedly wrapped around a cylinder.

Due to the high friction, its efficiency is so small that the ideal gain does not matter much. Depending on the direction of rise of the inclined plane, the screw thread can be left or right.
Examples of simple devices with screw threads are a jack, a bolt with a nut, a micrometer, a vise.

Traffic. Warmth Kitaygorodsky Alexander Isaakovich

Inclined plane

Inclined plane

A steep slope is more difficult to overcome than a gentle one. It is easier to roll a body to a height on an inclined plane than to lift it vertically. Why is that and how much easier? The law of the addition of forces allows us to understand these issues.

On fig. 12 shows a trolley on wheels, which is held on an inclined plane by the tension of a rope. In addition to traction, two more forces act on the cart - weight and the reaction force of the support, which always acts along the normal to the surface, regardless of whether the support surface is horizontal or inclined.

As already mentioned, if the body presses on the support, then the support counteracts the pressure or, as they say, creates a reaction force.

We are interested in the extent to which it is easier to pull the cart up an inclined plane than to lift it vertically.

Let us expand the forces so that one is directed along, and the other is perpendicular to the surface along which the body moves. In order for the body to rest on an inclined plane, the tensile force of the rope must balance only the longitudinal component. As for the second component, it is balanced by the reaction of the support.

Find the rope tension force of interest to us T can be either geometric construction or trigonometry. The geometric construction consists in drawing from the end of the weight vector P perpendicular to the plane.

In the figure, you can find two similar triangles. Inclined plane length ratio l to the height h is equal to the ratio of the corresponding sides in the triangle of forces. So,

The more sloping the inclined plane ( h/l small), so, of course, it is easier to drag the body up.

And now for those who know trigonometry: since the angle between the transverse component of the weight and the weight vector is equal to the angle? inclined plane (these are angles with mutually perpendicular sides), then

So, roll the cart on an inclined plane with an angle? in sin? times easier than lifting it vertically.

It is useful to remember the values ​​of trigonometric functions for angles of 30, 45 and 60°. Knowing these numbers for the sine (sin 30° = 1/2; sin 45° = sqrt(2)/2; *5 sin 60° = sqrt(3)/2), we get a good idea of ​​the gain in strength when moving along inclined plane.

From the formulas it can be seen that with an angle of an inclined plane of 30 °, our efforts will be half the weight: T = P(1/2). At angles of 45° and 60°, the rope will have to be pulled with forces equal to approximately 0.7 and 0.9 of the weight of the cart. As you can see, such steep inclined planes make things a little easier.